How do you explain an asymptote?
Table of Contents
- How do you explain an asymptote?
- What is an asymptote in real life?
- What does asymptote mean in psychology?
- Why do we use asymptotes?
- Why do we use Asymptotes?
- What are the three types of Asymptotes?
- Which is the best definition of the asymptote?
- When does a slant asymptote occur in math?
- When does the curve cross over the asymptote?
- Are there vertical asymptotes for X and Y?
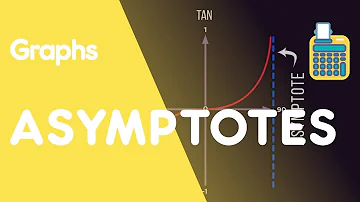
How do you explain an asymptote?
An asymptote is a line that a graph approaches without touching. Similarly, horizontal asymptotes occur because y can come close to a value, but can never equal that value. In the previous graph, there is no value of x for which y = 0 ( ≠ 0), but as x gets very large or very small, y comes close to 0.
What is an asymptote in real life?
The Application of an Asymptote in Real Life They are in use for significant O notations. They are simple approximations for complex equations. They are useful for graphing rational equations. They are relevant for- Algebra: Rational functions and Calculus: Limits of functions.
What does asymptote mean in psychology?
in psychology, the approach toward a full level of response or cure after many learning trials. ASYMPTOTE: "An asymptote represents a hypothetical straight line that a curve gets close to but never reaches as performance becomes more consistent. "
Why do we use asymptotes?
Asymptotes have a variety of applications: they are used in big O notation, they are simple approximations to complex equations, and they are useful for graphing rational equations. ... Typical examples would be ∞ and −∞, or the point where the denominator of a rational function equals zero.
Why do we use Asymptotes?
Asymptotes have a variety of applications: they are used in big O notation, they are simple approximations to complex equations, and they are useful for graphing rational equations. ... Typical examples would be ∞ and −∞, or the point where the denominator of a rational function equals zero.
What are the three types of Asymptotes?
There are three kinds of asymptotes: horizontal, vertical and oblique. For curves given by the graph of a function y = ƒ(x), horizontal asymptotes are horizontal lines that the graph of the function approaches as x tends to +∞ or −∞.
Which is the best definition of the asymptote?
Asymptote An asymptote is a line that a curve approaches, as it heads towards infinity:
When does a slant asymptote occur in math?
Slant Asymptotes. Sometimes, there is a slanted, or diagonal, line that a curve approaches but never quite reaches. One time this happens is when there is an x-squared in the numerator of a fraction and an x in the denominator.
When does the curve cross over the asymptote?
The curve can approach from any side (such as from above or below for a horizontal asymptote), or may actually cross over (possibly many times), and even move away and back again. The important point is that: The distance between the curve and the asymptote tends to zero as they head to infinity (or −infinity)
Are there vertical asymptotes for X and Y?
The y -values for these x -values does not exist on the graph. Hence, the two vertical asymptotes are x = 1 and x = -5. In fact, the value of y may go to positive or negative infinity as x approaches 1 or -5 along these vertical asymptotes.

 Main Topics
Main Topics


