Main TopicsHow do you prove a differential equation is exact?
Table of Contents
- How do you prove a differential equation is exact?
- What does it mean for a DE to be exact?
- What is exact and non exact differential equation?
- When a differential form is exact?
- Are all separable differential equations exact?
- How do you solve non exact equations?
- How do you solve exact de?
- Are all exact equations separable?
- What is exact solution?
- What do you mean by perfect differential?
- When is a given differential equation called an exact equation?
- How is an exact form of a differential called?
- Which is the exact equation of one variable?
- Which is an exact first order differential equation?
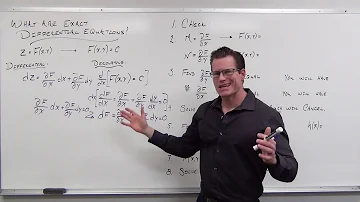
How do you prove a differential equation is exact?
Let us consider the equation P(x, y)dx + Q(x, y)dy equal to 0. Suppose that there exists a function v(x, y) such that dv = Mdx + Ndy, then the differential equation is said to be an exact differential equation solution is given by v(x, y) = c. Suppose that (1) is exact. Hence the given equation is exact.
What does it mean for a DE to be exact?
Therefore, if a differential equation has the form. for some function f( x, y), then it is automatically of the form df = 0, so the general solution is immediately given by f( x, y) = c. In this case, is called an exact differential, and the differential equation (*) is called an exact equation.
What is exact and non exact differential equation?
ο NON EXACT DIFFERENTIAL EQUATION β’ For the differential equation π π₯, π¦ ππ₯ + π π₯, π¦ ππ¦ = 0 IF ππ΄ ππ β ππ΅ ππ then, π«πππππππππππ π¬πππππππ ππ ππππ ππ ππ π΅πΆπ΅π¬πΏπ¨πͺπ» β’ If the given differential equation is not exact then make that equation exact by finding INTEGRATING FACTOR.
When a differential form is exact?
In mathematics, especially vector calculus and differential topology, a closed form is a differential form Ξ± whose exterior derivative is zero (dΞ± = 0), and an exact form is a differential form, Ξ±, that is the exterior derivative of another differential form Ξ².
Are all separable differential equations exact?
A first-order differential equation is exact if it has a conserved quantity. For example, separable equations are always exact, since by definition they are of the form: M(y)y + N(t)=0, ... so Ο(t, y) = A(y) + B(t) is a conserved quantity.
How do you solve non exact equations?
1:083:36How to solve non exact differential equations with an integrating factorYouTube
How do you solve exact de?
0:035:49Exact Differential Equations - YouTubeYouTube
Are all exact equations separable?
A first-order differential equation is exact if it has a conserved quantity. For example, separable equations are always exact, since by definition they are of the form: M(y)y + N(t)=0, ... How do we recognize whether a differential equation is exact, and how do we find a conserved quantity?
What is exact solution?
As used in physics, the term "exact" generally refers to a solution that captures the entire physics and mathematics of a problem as opposed to one that is approximate, perturbative, etc. Exact solutions therefore need not be closed-form.
What do you mean by perfect differential?
In multivariate calculus, a differential is said to be exact or perfect, as contrasted with an inexact differential, if it is of the form dQ, for some differentiable function Q.
When is a given differential equation called an exact equation?
for some function f ( x, y ), then it is automatically of the form df = 0, so the general solution is immediately given by f ( x, y) = c. In this case, is called an exact differential, and the differential equation (*) is called an exact equation. To determine whether a given differential equation
How is an exact form of a differential called?
An exact differential is sometimes also called a 'total differential', or a 'full differential', or, in the study of differential geometry, it is termed an exact form . Substituting the first equation into the second and rearranging, we obtain
Which is the exact equation of one variable?
A first-order differential equation (of one variable) is known as an exact, or an exact differential, if it is the result of a simple differentiation. The equation P(x, y)yβ² + Q(x, y) = 0, or in the equivalent alternate notation P(x, y)dy + Q(x, y)dx = 0, is exact if P x (x, y) = Q y (x, y).
Which is an exact first order differential equation?
A firstβorder differential equation is one that contains a firstβbut does not contain any higherβderivative of the unknown function. Once a differential equation M dx + N dy is equal to 0 is determined to be exact, the only task remaining is to find the function f (x, y) such that f x equals M and f y equals N. Q3.
